Our Flat Universe (the 'why' of shapes)

➤ Get a printable copy

Einstein’s theory of general relativity proposes that matter generates a gravitational field that warps the fabric of space-time. Does this mean that the entire universe naturally curved? There are three conceivable geometries for the universe.
Negative curvature: in this scenario, the universe adopts a saddle-like shape. On the surface, the angles of a triangle would add up to less than 180°.
Positive Curvature: In this scenario, the universe adopts a spherical form. On the surface of a sphere, the total angle sum exceeding 180°.
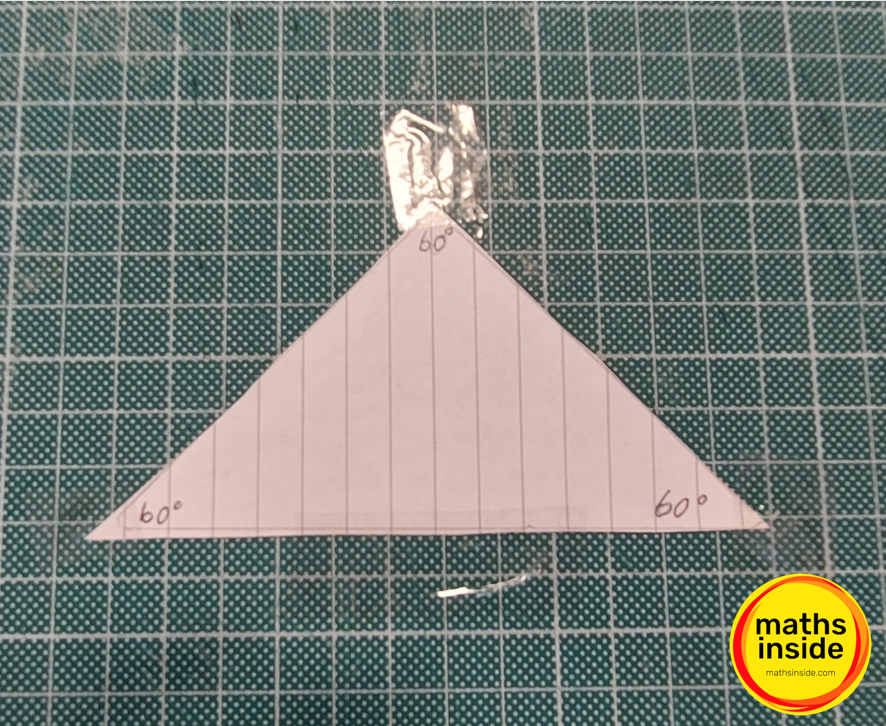
Flatness: In this case, the universe is entirely flat upon which the angles of a triangle sum up to precisely 180°.
Determining the universe’s geometry revolves around a crucial parameter known as ‘critical density’. This signifies the precise amount of matter required in the universe to maintain a flat configuration, avoiding excess expansion or collapse. The critical density relies on both the strength of gravity and the universes expansion rate, expressed as

Pcrit=(3Ho²/8πG)

Ho being the Hubble’s Constant.
G being the gravitational constant.

If the actual density of the universe is greater than that of the critical density, then the universe is spherical. If the actual density of the universe is lower than that of the critical density, then the universe is saddled-shaped. If the actual density of the universe is equal than that of the critical density, then the universe is flat. Cosmologists confidently assert that the universe is indeed flat because the actual density aligns so closely to the critical density.

— Callum Alexander (S6)